Reiter has suggested that the situations in the situation calculus be
defined axiomatically. He suggests the following four
axioms
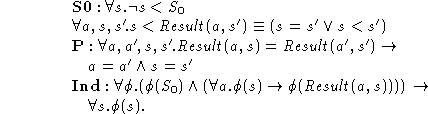
which determine equality of situations, relative to equality of events or actions. These axioms are categorical, that is relative to an interpretation of equality of actions, there is a unique model of situations.
Rather than use these axioms, which state that no other situations
exist between s and 
, we choose to say that situations
can be ordered by a < predicate, which is a strict partial order,
which we axiomatize as follows.
The predicate < is similar to the future(s,s') predicate,
introduced by [MH69], which is true when s' is in the future of
s. We find it useful to write this in infix notation, and to use 
as the non-strict version. It also is useful to write 
for 
